What is Force?

Definitions for Force:

1) Force is a push or pull

2) Force is the capacity to do work or cause physical change

3) Force= Mass times acceleration (F = ma)

4) A force is that which changes or tends to change the state of rest or motion of a body. 

Examples:

For simplicity sake, all forces (interactions) between objects can be placed into two broad categories: contact forces, and forces resulting from action-at-a-distance.

Contact Forces include: frictional forces, buoyant forces, normal forces, and air resistance forces 

Action-at-a-distance forces include: gravitation, electrostatic and magnetic forces.

Measuring Force:

Force is measured using either the English System of Measurements or the International System of Units (SI).

Common Units of Force

--->>SI: Newton (N) 1 N = 0.225 lb; 

One Newton (N) of force is defined as the amount of force needed to accelerate 1 kilogram (kg) of mass at a rate of 1 meter per second squared (m/s2).

1 Newton = 1 kg m/sec2 (A kilogram is the amount of weight at which 1 N of force will accelerate at a rate of 1 m/s2.)

--->>English System: Pound (LB) 1 LB = 4.448 N 

In English system of measurements, a slug is the amount of mass that 1 pound of force will accelerate at 1 ft/s2, and a pound mass is the amount of mass that 1 LB of force will accelerate at 32 feet/s2. 

Describing a Force:

A force is a vector quantity. A vector quantity is a quantity which has both magnitude and direction. To fully describe the force acting upon an object, you must describe both its magnitude and direction. Thus, 10 Newtons of force is not a complete description of the force acting on an object. 10 Newtons, downwards is a complete description of the force acting upon an object. 

(Note: What is the difference between vector and scalar quantities? A vector has both strength and direction, a scalar quantity can be described using only 1 quantity, magnitude. Examples of scalar quantities are: time, energy and volume since they only represent magnitude and no direction.

What is the Difference between Mass and Weight?

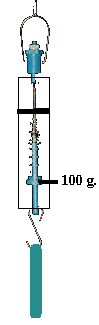
Shown below are two types of scales commonly used in the classroom --a spring scale (left) and a simple balance beam scale on the right.

On earth the spring scale reads 100g with an unknown mass attached at the bottom. To balance the scale on the right a 100g mass was also needed.

If we were to take both scales to the moon, what would the the spring scale read? How much mass would be needed to balance the 100g mass on the balance beam? Can you explain your answer? For more details see the Mass and Weight Page.

spring scale   simple balance scale What does applying a Force do? Force causes acceleration.  Newton's Second Law states that: the acceleration (a) of an object is directly proportional to the force (F) applied, and inversely proportional to the object's mass (m). That means that the more force you apply to an object, the greater the acceleration. And, the more mass the object has, the lower the acceleration.  Newton's Second Law can be written in equation form: F = ma.  For falling objects we can write F=mg where g is the acceleration due to gravity. The force of gravity is what causes free falling objects to accelerate. These objects all accelerate at the same rate of 9.8 meters/sec^2 What is Friction? Friction is the force that opposes the relative motion or tendency to such motion of two bodies in contact. If we try to push a block of wood across a table, there are two opposing forces that act: the force associated with the push, and a force that is associated with the friction which acts in the opposite direction. As frictional forces are decreased (for example, by placing oil on the table) the object moves further and further before stopping. This demonstrates Galileo's law of inertia which states: an object in a state of motion possesses an ``inertia'' that causes it to remain in that state of motion unless an external force acts on it. Try these exercise! Please enter your answer in the space provided: 1) How much net force is required to accelerate a 1500 kg car at 6.00 m/s2?  Please enter your answer in the space provided:   newtons 2) What is the mass of an object that has a weight of 115 N on the Moon? The gravity of the Moon is 1/6 of g (which is 9.8 m/s2).   kg.  3) An object in free fall will accelerate at a rate of :  m/sec/sec Multiple Choice Questions 1. Which of the following are contact forces?  a) magnetic forces  b) gravity  c) air resistance  d) electrostatic forces 2. Which of the following are action at a distance forces?  a) air resistance  b) normal forces  c) magnetic forces  d) frictional forces 3. What is the force that opposes the relative motion or tendency to such motion of two bodies in contact  a) magnetism  b) the normal force  c) gravity  d) friction 4. 1 kg-m/sec/sec is equal to-- a) 1 Newton  b) 1 pound  c) 1000 newtons  d) none of the above 5. Which of the following statements is not correct  a) air resistance is a force  b) mass is the result of the force of gravity  c) to accelerate an object requires a force  d) weight is the force of gravity